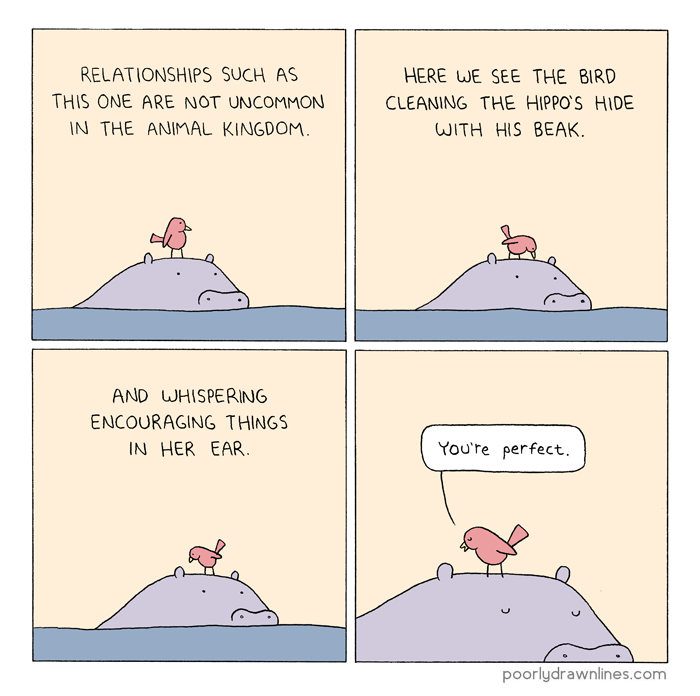
Shared posts
Linear Algebra on a Mission Impossible
I love making math questions out of the movies. Here is a Mission Impossible III question.
Tom Cruise is cute. He plays Ethan Hunt in Mission Impossible movies. In Mission Impossible III he needs to steal the Rabbit’s Foot from a secure skyscraper in Shanghai. He arrives in Shanghai and studies the skyscraper looking out his window. He decides to break in through the roof. And the way to get to the roof is to use a rope and swing across from another, even taller, skyscraper. 1:21 minutes into the movie, Ethan Hunt calculates the length of the rope he will need by using the projection of a skyline on his window, as seen on the first picture.

Explain why the projection is not enough to calculate the length of the rope. What other data does he need for that? Ethan Hunt does request extra data. But he makes one mistake. He uses his pencil as a compass to draw the end of the rope curve, as seen on the second picture. Explain what his mistake is.

p0kemina: I’m going to make a youtube video entitled "Shit ALL men say” and it will consist only of...
I’m going to make a youtube video entitled
"Shit ALL men say”
and it will consist only of the phrase “But not all men say that~!!”
And then I’ll wait for men to stare at their keyboards in utter distress as they contemplate the paradox of their intense desire and desperation to inform me that not all men say that.
I will break them.
The Mathematical Dialect Quiz
- What do you call a rigorous demonstration that a statement is true?
- If “proof,” then you’re a mathematician
- If “experiment,” then you’re a physicist
- If you have no word for this concept, then you’re an economist
- What do you call a slow, painful, computationally intense method of solving a problem?
- If “engineering,” then you’re a mathematician
- If “mathematics,” then you’re an engineer
- What do you call a person who is in their first job after a PhD?
- If “postdoc,” then you’re a mathematician or physicist
- If “assistant professor,” then you’re an economist
- If “wealthy,” then you’re a computer scientist
- If you have no word for a job after a PhD, then you’re in the humanities, and you have our condolences
- What do you call a calculator with graphing capabilities?

- How do you pronounce “Pythagorean”?
- If you pronounce it “pithAGorEan,” then you’re a mathematician
- If you pronounce it “PITHaGORean,” then you’re a physicist
- If you just mumble the word and hope no one notices, then you’re a TA
- What name do you use for the person who invented calculus?
- If “Leibniz,” then you’re a mathematician
- If “Newton,” then you’re a physicist
- If “magical wizard,” then you’re probably not ready for grad school
- What do you say after successfully proving your point beyond all doubt?
- If “QED,” then you’re a mathematician
- If “the prosecution rests,” then you’re a mathematician with a flair for drama
- If you do not believe proof beyond all doubt is possible, then you’re a scientist
- What do you call a simplified representation of reality, such as imagining a physical system with no friction or air resistance?
- If “a model,” then you’re a computer scientist
- If “an approximation,” then you’re an engineer
- If you call this “reality,” then you’re an economist
- How do you refer to a piece of work that suffers from one small but visible mistake?
- If “rough,” then you’re an engineer
- If “as good as it’s going to get,” then you’re a computer scientist
- If “worthless,” then you’re a mathematician
- What do you call a formal gathering of professionals from your field?
- If “a conference,” then you’re a physicist
- If “a start-up,” then you’re a computer scientist
- If “an advisory panel to the president,” then you’re an economist
- If “a game of D&D,” then you’re a mathematician
Thanks for reading! If you prefer bad gifs to bad drawings, you might also check out The Math Aficionado’s Guide to High Fives.
arXiv’s Police
I used to love arXiv. I’ve long thought that it was one of the best things that happened to mathematics. arXiv makes mathematical research available for free and without delay. Moreover, it is highly respected among mathematicians. For example, Grigori Perelman never submitted his proof of the Poincaré conjecture to any journal: he just posted it on arXiv.
When I came back to mathematics, all my math friends explained to me that I should submit my paper to arXiv on the same day that I submit it to a journal. As my trust in arXiv grew, I started submitting to arXiv first, waiting one week for comments, and then submitting to a journal.
Now it seems that arXiv might not love its contributors as much as they used to. arXiv moderators seem to be getting harsher and harsher. Here is my story.
In June of 2013, I submitted my paper “A Line of Sages” to arXiv. This paper is about a new hat puzzle that appeared at the Tournaments of the Towns in March 2013. The puzzle was available online at the Tournaments of the Towns webpage in Russian. After some thought I decided that it is better to cite the Tournament itself inside the body of the paper, rather than to have a proper reference. Online references in general are not stable, and this particular one was in Russian. Very soon this competition will be translated into English and the puzzle will appear in all standard math competition archives.
arXiv rejected my paper. A moderator complained that I didn’t have a bibliography. So I created a bibliography with the link to the puzzle. My paper was rejected again saying that the link wasn’t stable. Duh. That’s the reason why I didn’t put it there from the start. I Goggled the puzzle and I still didn’t find any other links.
I argued with my moderator that the standards for papers in recreational mathematics are different from the standards for purely research papers. Short recreational notes do not require two pages of history and background, nor a long list of references. A recreational paper doesn’t need to have theorems and lemmas.
Meanwhile, the moderator complained that the paper was “not sufficiently motivated to be interesting to the readership.”
I got tired of exchanging emails with this moderator and submitted my paper to The Mathematical Intelligencer, where it was immediately accepted. So I dropped my submission to arXiv.
Now my paper is not available for free on arXiv. But anyone can freely buy it from Springer for $39.95.
Minas Gerais: Prioridades.

Minas Gerais: Prioridades.
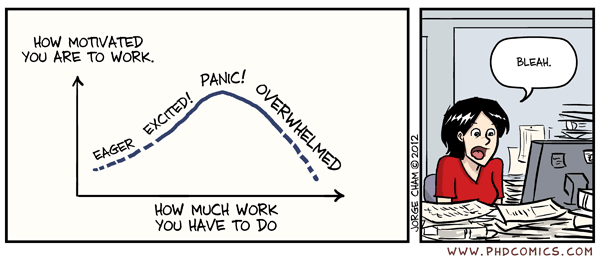
11/1/13 PHD comic: 'Peak Panic'
| Piled Higher & Deeper by Jorge Cham |
www.phdcomics.com
|
|
 |
||
|
title:
"Peak Panic" - originally published
11/1/2013
For the latest news in PHD Comics, CLICK HERE! |
||
Letter from a Chinese forced-labor camp found in Kmart Hallowe'en decorations

Since last Hallowe'en, a woman in Oregon has been circulating a letter she found in a box of decorative tombstones she bought at Kmart. The letter was written by a prisoner in a forced labor camp in China's Masanjia camp; he was imprisoned for practicing Falun Gong, a banned religion whose members have long been targetted for brutal suppression by the Chinese state. CNN located the ex-prisoner and interviewed him as he narrated a story of "inhumane torture" at the camp.
Inside The Chinese Labor Camp That Made Halloween Decorations Sold At Kmart [Laura Northrup/Consumerist]Their staff in Beijing spent months searching for the man. Finally, they found him and confirmed his identity, but didn’t reveal it to the public. He is a follower of Falun Gong, which most of the world calls a Buddhist-Taoist spiritual movement but the Chinese government considers a dangerous cult. He was sentenced to two and a half years in the Masanjia labor camp.
He reports sleep deprivation, beatings, and other misery in the labor camp. Making Halloween decorations for no pay was actually a reprieve for the inmates. Still, he decided to send a total of six letters, somehow procuring two items that inmates weren’t supposed to have: paper (taken from a re-education workbook) and a pen. He wrote the letter in bed, in the dark, avoiding the gaze of the guard who watched everyone while they slept. You know, to make sure they weren’t doing anything subversive like sending letters to the West in the products they were packaging.

![]()
Polícia de SP matou 41% menos neste ano porque foi proibida de prestar "socorro" no local
 |
submitted by BecomingFree [link] [10 comments] |
Don't Fly During Ramadan
A couple of weeks ago, I was scheduled to take a trip from New York (JFK) to Los Angeles on JetBlue. Every year, my family goes on a one-week pilgrimage, where we put our work on hold and spend time visiting temples, praying, and spending time with family and friends. To my Jewish friends, I often explain this trip as vaguely similar to the Sabbath, except we take one week of rest per year, rather than one day per week.
Our family is not Muslim, but by coincidence, this year, our trip happened to be during the last week of Ramadan.
By further coincidence, this was also the same week that I was moving out of my employer-provided temporary housing (at NYU) and moving into my new apartment. The night before my trip, I enlisted the help of two friends and we took most of my belongings, in a couple of suitcases, to my new apartment. The apartment was almost completely unfurnished - I planned on getting new furniture upon my return - so I dropped my few bags (one containing an air mattress) in the corner. Even though I hadn’t decorated the apartment yet, in accordance with Hindu custom, I taped a single photograph to the wall in my bedroom — a long-haired saint with his hands outstretched in pronam (a sign of reverence and respect).
The next morning, I packed the rest of my clothes into a suitcase and took a cab to the airport. I didn’t bother to eat breakfast, figuring I would grab some yogurt in the terminal while waiting to board.
I got in line for security at the airport and handed the agent my ID. Another agent came over and handed me a paper slip, which he said was being used to track the length of the security lines. He said, “just hand this to someone when your stuff goes through the x-ray machines, and we’ll know how long you were in line.’ I looked at the timestamp on the paper: 10:40.
When going through the security line, I opted out (as I always used to) of the millimeter wave detectors. I fly often enough, and have opted out often enough, that I was prepared for what comes next: a firm pat-down by a TSA employee wearing non-latex gloves, who uses the back of his hand when patting down the inside of the thighs.
After the pat-down, the TSA agent swabbed his hands with some cotton-like material and put the swab in the machine that supposedly checks for explosive residue. The machine beeped. “We’re going to need to pat you down again, this time in private,” the agent said.
Having been selected before for so-called “random” checks, I assumed that this was another such check.
"What do you mean, ‘in private’? Can’t we just do this out here?"
"No, this is a different kind of pat-down, and we can’t do that in public." When I asked him why this pat-down was different, he wouldn’t tell me. When I asked him specifically why he couldn’t do it in public, he said "Because it would be obscene."
Naturally, I balked at the thought of going somewhere behind closed doors where a person I just met was going to touch me in “obscene” ways. I didn’t know at the time (and the agent never bothered to tell me) that the TSA has a policy that requires two agents to be present during every private pat-down. I’m not sure if that would make me feel more or less comfortable.
Noticing my hesitation, the agent offered to have his supervisor explain the procedure in more detail. He brought over his supervisor, a rather harried man who, instead of explaining the pat-down to me, rather rudely explained to me that I could either submit immediately to a pat-down behind closed-doors, or he could call the police.
At this point, I didn’t mind having to leave the secure area and go back through security again (this time not opting out of the machines), but I didn’t particularly want to get the cops involved. I told him, “Okay, fine, I’ll leave”.
"You can’t leave here."
"Are you detaining me, then?" I’ve been through enough "know your rights" training to know how to handle police searches; however, TSA agents are not law enforcement officials. Technically, they don’t even have the right to detain you against your will.
"We’re not detaining you. You just can’t leave." My jaw dropped.
"Either you’re detaining me, or I’m free to go. Which one is it?" I asked.
He glanced for a moment at my backpack, then snatched it out of the conveyor belt. “Okay,” he said. “You can leave, but I’m keeping your bag.”
I was speechless. My bag had both my work computer and my personal computer in it. The only way for me to get it back from him would be to snatch it back, at which point he could simply claim that I had assaulted him. I was trapped.
While we waited for the police to arrive, I took my phone and quickly tried to call my parents to let them know what was happening. Unfortunately, my mom’s voicemail was full, and my dad had never even set his up.
"Hey, what’s he doing?" One of the TSA agents had noticed I was touching my phone. "It’s probably fine; he’s leaving anyway," another said.
The cops arrived a few minutes later, spoke with the TSA agents for a moment, and then came over and gave me one last chance to submit to the private examination. “Otherwise, we have to escort you out of the building.” I asked him if he could be present while the TSA agent was patting me down.
"No," he explained, "because when we pat people down, it’s to lock them up."
I only realized the significance of that explanation later. At this point, I didn’t particularly want to miss my flight. Foolishly, I said, “Fine, I’ll do it.”
The TSA agents and police escorted me to a holding room, where they patted me down again - this time using the front of their hands as they passed down the front of my pants. While they patted me down, they asked me some basic questions.
"What’s the purpose of your travel?"
"Personal," I responded, (as opposed to business).
"Are you traveling with anybody?"
"My parents are on their way to LA right now; I’m meeting them there."
"How long is your trip?"
"Ten days."
"What will you be doing?"
Mentally, I sighed. There wasn’t any other way I could answer this next question.
"We’ll be visiting some temples." He raised his eyebrow, and I explained that the next week was a religious holiday, and that I was traveling to LA to observe it with my family.
After patting me down, they swabbed not only their hands, but also my backpack, shoes, wallet, and belongings, and then walked out of the room to put it through the machine again. After more than five minutes, I started to wonder why they hadn’t said anything, so I asked the police officer who was guarding the door. He called over the TSA agent, who told me,
"You’re still setting off the alarm. We need to call the explosives specialist".
I waited for about ten minutes before the specialist showed up. He walked in without a word, grabbed the bins with my possessions, and started to leave. Unlike the other agents I’d seen, he wasn’t wearing a uniform, so I was a bit taken aback.
"What’s happening?" I asked.
"I’m running it through the x-ray again," he snapped. "Because I can. And I’m going to do it again, and again, until I decide I’m done". He then asked the TSA agents whether they had patted me down. They said they had, and he just said, "Well, try again", and left the room. Again I was told to stand with my legs apart and my hands extended horizontally while they patted me down all over before stepping outside.
The explosives specialist walked back into the room and asked me why my clothes were testing positive for explosives. I told him, quite truthfully, “I don’t know.” He asked me what I had done earlier in the day.
"Well, I had to pack my suitcase, and also clean my apartment."
"And yesterday?"
"I moved my stuff from my old apartment to my new one".
"What did you eat this morning?"
"Nothing," I said. Only later did I realize that this made it sound like I was fasting, when in reality, I just hadn’t had breakfast yet.
"Are you taking any medications?"
The other TSA agents stood and listened while the explosives specialist and asked every medication I had taken “recently”, both prescription and over-the-counter, and asked me to explain any medical conditions for which any prescription medicine had been prescribed. Even though I wasn’t carrying any medication on me, he still asked for my complete “recent” medical history.
"What have you touched that would cause you to test positive for certain explosives?"
"I can’t think of anything. What does it say is triggering the alarm?" I asked.
"I’m not going to tell you! It’s right here on my sheet, but I don’t have to tell you what it is!" he exclaimed, pointing at his clipboard.
I was at a loss for words. The first thing that came to my mind was, “Well, I haven’t touched any explosives, but if I don’t even know what chemical we’re talking about, I don’t know how to figure out why the tests are picking it up.”
He didn’t like this answer, so he told them to run my belongings through the x-ray machine and pat me down again, then left the room.
I glanced at my watch. Boarding would start in fifteen minutes, and I hadn’t even had anything to eat. A TSA officer in the room noticed me craning my neck to look at my watch on the table, and he said, “Don’t worry, they’ll hold the flight.”
As they patted me down for the fourth time, a female TSA agent asked me for my baggage claim ticket. I handed it to her, and she told me that a woman from JetBlue corporate security needed to ask me some questions as well. I was a bit surprised, but agreed. After the pat-down, the JetBlue representative walked in and cooly introduced herself by name.
She explained, “We have some questions for you to determine whether or not you’re permitted to fly today. Have you flown on JetBlue before?”
"Yes"
"How often?"
"Maybe about ten times," I guessed.
"Ten what? Per month?"
"No, ten times total."
She paused, then asked,
"Will you have any trouble following the instructions of the crew and flight attendants on board the flight?"
"No." I had no idea why this would even be in doubt.
"We have some female flight attendants. Would you be able to follow their instructions?"
I was almost insulted by the question, but I answered calmly, “Yes, I can do that.”
"Okay," she continued, "and will you need any special treatment during your flight? Do you need a special place to pray on board the aircraft?"
Only here did it hit me.
"No," I said with a light-hearted chuckle, trying to conceal any sign of how offensive her questions were. "Thank you for asking, but I don’t need any special treatment."
She left the room, again, leaving me alone for another ten minutes or so. When she finally returned, she told me that I had passed the TSA’s inspection. “However, based on the responses you’ve given to questions, we’re not going to permit you to fly today.”
I was shocked. “What do you mean?” were the only words I could get out.
"If you’d like, we’ll rebook you for the flight tomorrow, but you can’t take the flight this afternoon, and we’re not permitting you to rebook for any flight today."
I barely noticed the irony of the situation - that the TSA and NYPD were clearing me for takeoff, but JetBlue had decided to ground me. At this point, I could think of nothing else but how to inform my family, who were expecting me to be on the other side of the country, that I wouldn’t be meeting them for dinner after all. In the meantime, an officer entered the room and told me to continue waiting there. “We just have one more person who needs to speak with you before you go.” By then, I had already been “cleared” by the TSA and NYPD, so I couldn’t figure out why I still needed to be questioned. I asked them if I could use my phone and call my family.
"No, this will just take a couple of minutes and you’ll be on your way." The time was 12.35.
He stepped out of the room - for the first time since I had been brought into the cell, there was no NYPD officer guarding the door. Recognizing my short window of opportunity, I grabbed my phone from the table and quickly texted three of my local friends - two who live in Brooklyn, and one who lives in Nassau County - telling them that I had been detained by the TSA and that I couldn’t board my flight. I wasn’t sure what was going to happen next, but since nobody had any intention of reading me my Miranda rights, I wanted to make sure people knew where I was.
After fifteen minutes, one of the police officers marched into the room and scolded, “You didn’t tell us you have a checked bag!” I explained that I had already handed my baggage claim ticket to a TSA agent, so I had in fact informed someone that I had a checked bag. Looking frustrated, he turned and walked out of the room, without saying anything more.
After about twenty minutes, another man walked in and introduced himself as representing the FBI. He asked me many of the same questions I had already answered multiple times - my name, my address, what I had done so far that day. etc.
He then asked, “What is your religion?”
"I’m Hindu."
"How religious are you? Would you describe yourself as ‘somewhat religious’ or ‘very religious’?"
I was speechless from the idea of being forced to talk about my the extent of religious beliefs to a complete stranger. “Somewhat religious”, I responded.
"How many times a day do you pray?" he asked. This time, my surprise must have registered on my face, because he quickly added, "I’m not trying to offend you; I just don’t know anything about Hinduism. For example, I know that people are fasting for Ramadan right now, but I don’t have any idea what Hindus actually do on a daily basis."
I nearly laughed at the idea of being questioned by a man who was able to admit his own ignorance on the subject matter, but I knew enough to restrain myself. The questioning continued for another few minutes. At one point, he asked me what cleaning supplies I had used that morning.
"Well, some window cleaner, disinfectant -" I started, before he cut me off.
"This is important," he said, sternly. "Be specific." I listed the specific brands that I had used.
Suddenly I remembered something: the very last thing I had done before leaving was to take the bed sheets off of my bed, as I was moving out. Since this was a dorm room, to guard against bedbugs, my dad (a physician) had given me an over-the-counter spray to spray on the mattress when I moved in, over two months previously. Was it possible that that was still active and triggering their machines?
"I also have a bedbug spray," I said. "I don’t know the name of it, but I knew it was over-the-counter, so I figured it probably contained permethrin." Permethrin is an insecticide, sold over-the-counter to kill bed bugs and lice.
"Perm-what?" He asked me to spell it.
After he wrote it down, I asked him if I could have something to drink. “I’ve been here talking for three hours at this point,” I explained. “My mouth is like sandpaper”. He refused, saying
"We’ll just be a few minutes, and then you’ll be able to go."
"Do you have any identification?" I showed him my drivers license, which still listed my old address. "You have nothing that shows your new address?” he exclaimed.
"Well, no, I only moved there on Thursday."
"What about the address before that?"
"I was only there for two months - it was temporary housing for work". I pulled my NYU ID out of my wallet. He looked at it, then a police officer in the room took it from him and walked out.
"What about any business cards that show your work address?" I mentally replayed my steps from the morning, and remembered that I had left behind my business card holder, thinking I wouldn’t need it on my trip.
"No, I left those at home."
"You have none?”
"Well, no, I’m going on vacation, so I didn’t refill them last night." He scoffed. "I always carry my cards on me, even when I’m on vacation." I had no response to that - what could I say?
"What about a direct line at work? Is there a phone number I can call where it’ll patch me straight through to your voicemail?"
"No," I tried in vain to explain. "We’re a tech company; everyone just uses their cell phones". To this day, I don’t think my company has a working landline phone in the entire office - our "main line" is a virtual assistant that just forwards calls to our cell phones. I offered to give him the name and phone number of one of our venture partners instead, which he reluctantly accepted.
Around this point, the officer who had taken my NYU ID stormed into the room.
"They put an expiration sticker on your ID, right?" I nodded. "Well then why did this ID expire in 2010?!" he accused.
I took a look at the ID and calmly pointed out that it said “August 2013” in big letters on the ID, and that the numbers “8/10” meant “August 10th, 2013”, not “August, 2010”. I added, “See, even the expiration sticker says 2013 on it above the date”. He studied the ID again for a moment, then walked out of the room again, looking a little embarrassed.
The FBI agent resumed speaking with me. “Do you have any credit cards with your name on them?” I was hesitant to hand them a credit card, but I didn’t have much of a choice. Reluctantly, I pulled out a credit card and handed it to him. “What’s the limit on it?” he said, and then, noticing that I didn’t laugh, quickly added, “That was a joke.”
He left the room, and then a series of other NYPD and TSA agents came in and started questioning me, one after the other, with the same questions that I’d already answered previously. In between, I was left alone, except for the officer guarding the door.
At one point, when I went to the door and asked the officer when I could finally get something to drink, he told me, “Just a couple more minutes. You’ll be out of here soon.”
"That’s what they said an hour ago," I complained.
"You also said a lot of things, kid," he said with a wink. "Now sit back down".
I sat back down and waited some more. Another time, I looked up and noticed that a different officer was guarding the door. By this time, I hadn’t had any food or water in almost eighteen hours. I could feel the energy draining from me, both physically and mentally, and my head was starting to spin. I went to the door and explained the situation the officer. “At the very least, I really need something to drink.”
"Is this a medical emergency? Are you going to pass out? Do we need to call an ambulance?" he asked, skeptically. His tone was almost mocking, conveying more scorn than actual concern or interest.
"No," I responded. I’m not sure why I said that. I was lightheaded enough that I certainly felt like I was going to pass out.
"Are you diabetic?"
"No," I responded.
Again he repeated the familiar refrain. “We’ll get you out of here in a few minutes.” I sat back down. I was starting to feel cold, even though I was sweating - the same way I often feel when a fever is coming on. But when I put my hand to my forehead, I felt fine.
One of the police officers who questioned me about my job was less-than-familiar with the technology field.
"What type of work do you do?"
"I work in venture capital."
"Venture Capital - is that the thing I see ads for on TV all the time?" For a moment, I was dumbfounded - what venture capital firm advertises on TV? Suddenly, it hit me.
"Oh! You’re probably thinking of Capital One Venture credit cards." I said this politely and with a straight face, but unfortunately, the other cop standing in the room burst out laughing immediately. Silently, I was shocked - somehow, this was the interrogation procedure for confirming that I actually had the job I claimed to have.
Another pair of NYPD officers walked in, and one asked me to identify some landmarks around my new apartment. One was, “When you’re facing the apartment, is the parking on the left or on the right?” I thought this was an odd question, but I answered it correctly. He whispered something in the ear of the other officer, and they both walked out.
The onslaught of NYPD agents was broken when a South Asian man with a Homeland Security badge walked in and said something that sounded unintelligible. After a second, I realized he was speaking Hindi.
"Sorry, I don’t speak Hindi."
"Oh!" he said, noticeably surprised at how "Americanized" this suspect was. We chatted for a few moments, during which time I learned that his family was Pakistani, and that he was Muslim, though he was not fasting for Ramadan. He asked me the standard repertoire of questions that I had been answering for other agents all day.
Finally, the FBI agent returned.
"How are you feeling right now?" he asked. I wasn’t sure if he was expressing genuine concern or interrogating me further, but by this point, I had very little energy left.
"A bit nauseous, and very thirsty."
"You’ll have to understand, when a person of your… background walks into here, travelling alone, and sets off our alarms, people start to get a bit nervous. I’m sure you’ve been following what’s been going on in the news recently. You’ve got people from five different branches of government all in here - we don’t do this just for fun."
He asked me to repeat some answers to questions that he’d asked me previously, looking down at his notes the whole time, then he left. Finally, two TSA agents entered the room and told me that my checked bag was outside, and that I would be escorted out to the ticketing desks, where I could see if JetBlue would refund my flight.
It was 2:20PM by the time I was finally released from custody. My entire body was shaking uncontrollably, as if I were extremely cold, even though I wasn’t. I couldn’t identify the emotion I was feeling. Surprisingly, as far as I could tell, I was shaking out of neither fear nor anger - I felt neither of those emotions at the time. The shaking motion was entirely involuntary, and I couldn’t force my limbs to be still, no matter how hard I concentrated.
In the end, JetBlue did refund my flight, but they cancelled my entire round-trip ticket. Because I had to rebook on another airline that same day, it ended up costing me about $700 more for the entire trip. Ironically, when I went to the other terminal, I was able to get through security (by walking through the millimeter wave machines) with no problem.
I spent the week in LA, where I was able to tell my family and friends about the entire ordeal. They were appalled by the treatment I had received, but happy to see me safely with them, even if several hours later.
I wish I could say that the story ended there. It almost did. I had no trouble flying back to NYC on a red-eye the next week, in the wee hours of August 12th. But when I returned home the next week, opened the door to my new apartment, and looked around the room, I couldn’t help but notice that one of the suitcases sat several inches away from the wall. I could have sworn I pushed everything to the side of the room when I left, but I told myself that I may have just forgotten, since I was in a hurry when I dropped my bags off.
When I entered my bedroom, a chill went down my spine: the photograph on my wall had vanished. I looked around the room, but in vain. My apartment was almost completely empty; there was no wardrobe it could have slipped under, even on the off-chance it had fallen.
To this day, that photograph has not turned up. I can’t think of any “rational” explanation for it. Maybe there is one. Maybe a burglar broke into my apartment by picking the front door lock and, finding nothing of monetary value, took only my picture. In order to preserve my peace-of-mind, I’ve tried to convince myself that that’s what happened, so I can sleep comfortably at night.
But no matter how I’ve tried to rationalize this in the last week and a half, nothing can block out the memory of the chilling sensation I felt that first morning, lying on my air mattress, trying to forget the image of large, uniformed men invading the sanctuary of my home in my absence, wondering when they had done it, wondering why they had done it.
In all my life, I have only felt that same chilling terror once before - on one cold night in September twelve years ago, when I huddled in bed and tried to forget the terrible events in the news that day, wondering why they they had happened, wondering whether everything would be okay ever again.
1. Go to Youtube and select a video. This one is perfect. 2....
1. Go to Youtube and select a video. This one is perfect.
2. Pause the video.
3. Click outside the video area and type 1980.
4. ???
5. Profit
MIT Uses Machine Learning Algorithm To Make TCP Twice As Fast
Read more of this story at Slashdot.
Cohomology Detects Failures of Classical Mathematics
Ordinarily I try to avoid cross-posting between here and the Homotopy Type Theory blog, but this remark seems probably of interest to the crowd here as well. After my recent post there about how to define cohomology in HoTT, Zhen Lin kindly pointed me to a very nice paper of Andreas Blass called Cohomology detects failures of the axiom of choice. A main result of this paper is that the following two statements are equivalent (over ZF):
- The axiom of choice.
- For any discrete set X and any (not necessarily abelian) group G, the cohomology H1(X;G) vanishes.
The fact that discrete spaces have no higher cohomology is “obvious” in classical algebraic topology, but this shows that if we want to do homotopy theory “constructively” then we need to give up that expectation. In this post I want to explain one of Blass’s proofs from a geometric point of view, and then consider what cohomology has to say about the law of excluded middle.
Here is one of Blass’s proofs that the vanishing of the cohomology of all sets implies AC. Let p:Y→X be a surjection of sets, for which we want to find a section.
Suppose first that every fiber Yx=p−1(x) is isomorphic to some fixed nonempty set S. Of course, if we were given specified isomorphisms ϕx:S≅Yx for all x, then we could fix a particular element s∈S and define f:X→Y by f(x)=ϕx(s), but we are only assuming that such isomorphisms exist. (In HoTT language, they “merely” exist.)
In this special case, from a geometric point of view, we can consider Y as a locally trivial bundle over X with fiber S, and we know that such bundles are classified by the cohomology of the base with coefficients in the automorphism group of the fiber. Thus, if we let G=Aut(S), we should be able to construct a “characteristic class” of Y in H1(X;G).
The way to do this depends on the definition of H1. Blass points out that many of the classical definitions are not correct in the absence of choice (his criterion of “correctness” being that they give rise to long exact sequences). He takes the route of simply defining H1(X;G) to be the set of isomorphism classes of G-torsors over X.
From a category-theoretic (or HoTT) point of view, it’s more natural to define H1(X;G) to be the set of natural isomorphism classes of functors X→BG, where BG is the one-object groupoid corresponding to G. It’s again easy to construct our characteristic class in this case, since for G=Aut(S), the groupoid BG is equivalent to the groupoid B′G, whose objects are sets isomorphic to S and whose morphisms are all isomorphisms between them. Then x↦Yx defines a functor from X to B′G, hence also to BG.
(In ZF, this equivalence of groupoids is only a “weak equivalence”, i.e. there is a functor BG→B′G that is essentially surjective and fully faithful. Thus, our “functor” X→BG is only an anafunctor, so we have to use those in the definition of cohomology. In HoTT, however, this is not a problem.)
Now by assumption, H1(X;G)=0, so this characteristic class is trivial. This means that our functor Y:X→B′G is naturally isomorphic to the one that is constant at S. Such a natural transformation assigns to each x∈X an isomorphism S≅Yx, and as we remarked above this is sufficient to define a section of p.
This handles the special case when all the fibers of p are isomorphic. In order to deal with the general case, Blass uses a trick: he defines U to be the set of nonempty finite sequences of elements of Y, with q:U→X the function which selects the first element of a sequence and then maps it down to X via p. Then the fiber Ux is a subset of U, but there also exists an injection U↪Ux that adjoins some fixed element of Yx at the front of each sequence. By the Schroeder-Bernstein theorem, therefore, U≅Ux, and so we can apply the previous case to get a section of q, and hence (by selecting first elements) a section of p.
It’s important, of course, that the Schroeder-Bernstein theorem does not depend on the axiom of choice. It does, however, depend on the law of excluded middle, so we might wonder, is Blass’s theorem still valid constructively?
Now by a classical result of Bishop and Diaconescu, the axiom of choice implies the law of excluded middle. I will explain the proof in a moment; the crucial thing to note is that it only uses AC to obtain a section of a surjection all of whose fibers are isomorphic. Since the first part of Blass’s argument (when all fibers are isomorphic) seems perfectly constructive, it will therefore also imply LEM, so that the second part of the argument can freely use Schroeder-Bernstein.
Why does AC imply LEM? Suppose P is a proposition for which we want to show “P or not P”. Let ΣP be the quotient of 2={N,S} by the equivalence relation which identifies N∼S exactly when P holds. (In reproducing this proof in HoTT, Spitters and Rijke have observed that ΣP is exactly the homotopy-theoretic suspension of P, hence the notation.) Then p:2→ΣP is a surjection. If it has a section f:ΣP→2, then we can consider f([N]) and f([S]), each of which is either N or S. This gives four cases, two of which easily imply P, and the other two of which easily imply “not P”.
Now what are the fibers of p? Every element of ΣP is either [N] or [S] (though this is not necessarily an exclusive-or), so it suffices to show that the fibers over [N] and [S] are isomorphic. The fiber over [N] always contains N, and it contains S iff P. Similarly, the fiber over [S] always contains S, and it contains N iff P. Thus, the two are isomorphic by an isomorphism that interchanges N and S.
Thus we recover, even constructively, Blass’s theorem that the vanishing of H1 of sets is equivalent to the axiom of choice. Blass’s original theorem was actually sharper: the vanishing of H1(X;G) for all G and a particular set X is equivalent to projectivity of X. Can this sharper version also be obtained constructively? I don’t know.
Regardless, I think it’s quite cute that we can connect geometric ideas like characteristic classes of fiber bundles in cohomology with logical ideas like the axiom of choice. In some sense, this is what topos theory is supposed to be all about.
Cantor’s theorem, the prisoner’s dilemma, and the halting problem
Cantor’s theorem is somewhat infamous as a mathematical result that many non-mathematicians have a hard time believing. Trying to disprove Cantor’s theorem is a popular hobby among students and cranks; even Eliezer Yudkowsky1993 fell into this trap once. I think part of the reason is that the standard proof is not very transparent, and consequently is hard to absorb on a gut level.
The goal of this post is to present a rephrasing of the statement and proof of Cantor’s theorem so that it is no longer about sets, but about a particular kind of game related to the prisoner’s dilemma. Rather than showing that there are no surjections , we will show that a particular kind of player in this game can’t exist. This rephrasing may make the proof more transparent and easier to absorb, although it will take some background material about the prisoner’s dilemma to motivate. As a bonus, we will almost by accident run into a proof of the undecidability of the halting problem.
Cantor’s theorem
If are two sets, let
denote the set of functions
. In particular, we will write the power set of
as
, where
, since we can identify a subset
of
with its indicator function
.
Theorem: Let be a set. Then there does not exist a surjection
.
Proof. Suppose otherwise, and let be a surjection. Consider the subset
.
By hypothesis, there exists such that
. But now if
, then
, so
, but if
, then
, so
; contradiction. Hence
does not exist.
Note that the proof goes through perfectly fine for a finite set, where it tells us that
for all non-negative integers
. This is one hint that Cantor’s theorem is not really about infinite sets, even though its main application is proving that infinite sets come in different sizes.
The prisoner’s dilemma
A prisoner’s dilemma is any two-player game in which the players move simultaneously; each player has two possible moves, cooperate or defect; and each player prefers outcomes in the following order:
- (I defect, you cooperate), often called exploiting the other player, followed by
- (I cooperate, you cooperate), then
- (I defect, you defect), and then
- (I cooperate, you defect), often called being exploited.
A common way of analyzing games like the prisoner’s dilemma is to determine their Nash equilibria. To define these, we first need the notion of a mixed strategy, which is a probability distribution over possible moves. A Nash equilibria in a game is then a choice of mixed strategy for each player such that each player, upon learning the mixed strategy of the other player, does not wish to change their strategy. Nash famously proved that in a game where each player has finitely many possible moves, a Nash equilibria always exists. We need to consider mixed rather than pure strategies because Nash equilibria are mixed in general; for example, the unique Nash equilibrium in rock-paper-scissors is that each player chooses rock, paper, or scissors with probability each.
The prisoner’s dilemma roughly models many real-world situations, and is of interest because the only Nash equilibrium in the game is that both players defect (since both players, upon learning what move their opponent is making, would strictly prefer to change their move to defect), but this situation is worse for both players than if both players had cooperated. This raises some interesting questions about how mutual cooperation can occur among rational agents of the kind considered in game theory, and possibly also some questions about how mutual cooperation can evolve in nature.
One idea is to consider the iterated prisoner’s dilemma, where two players repeatedly engage in a prisoner’s dilemma for many rounds. Here it is important to assign point values to the various outcomes and specify that both players are trying to maximize the number of points they have, and then it could be a sensible strategy to cooperate rather than defect in the hopes of promoting cooperation on future rounds. Unlike a one-shot prisoner’s dilemma (one that lasts one round) where it is assumed that you don’t know anything about your opponent, in an iterated prisoner’s dilemma you and your opponent learn about each other over time, so if you each learn that the other is cooperative then mutual cooperation might occur.
Axelrod famously ran an iterated prisoner’s dilemma tournament played by programs implementing various strategies, and found empirically that greedy strategies (that try to exploit opponents) generally did worse than altruistic strategies (that offered cooperation even with the possibility of being exploited). The most successful deterministic such strategy was tit for tat, which is also very simple to describe: cooperate on the first round, then repeat whatever your opponent did on the previous round. Tit for tat achieves mutual cooperation against other players that also cooperate but immediately attempts to punish defection with defection of its own. The iterated prisoner’s dilemma is a plausible rough model of social interaction, and tit for tat (or its more altruistic cousin, generous tit for tat, which occasionally forgives defection on the part of its opponent), is a plausible rough model of how cooperation among social animals like humans works.
But what about trying to achieve mutual cooperation on the one-shot prisoner’s dilemma? Unlike the iterated prisoner’s dilemma, it seems that you have no chance to learn anything about your opponent. Here a standard argument makes an additional assumption: namely, that you assume your opponent reasons the same way you do and that you both have common knowledge of this. (It is also typical to further assume that the payoff matrix is symmetric.) In this case, it seems plausible that if you cooperate, then so will your opponent, and similarly for defection. In other words, exploitation is asymmetric and therefore seems to be ruled out, and in that case you should cooperate expecting your opponent to have come to the same conclusion. In the philosophy literature this is known as the symmetry argument for cooperation; Hofstadter calls this superrationality.
However, this argument is philosophically contentious. It almost seems to be claiming that choosing to cooperate would somehow cause your opponent to cooperate, while choosing to defect would cause your opponent to defect, which seems implausible. The contention here is related to issues surrounding Newcomb’s problem and decision theory which we’ll avoid for the time being. Can we say something less contentious?
The prisoner’s dilemma with visible source code
One reason the iterated prisoner’s dilemma is different from the one-shot prisoner’s dilemma is that playing multiple rounds allows you to learn about your opponent. Consider the following alternate setup for learning about your opponent while only playing one round: two players, rather than play against each other directly, write programs to play a one-shot prisoner’s dilemma for them. Moreover, the programs are fed as input each other’s source code. Call this the prisoner’s dilemma with visible source code. For a brief introduction to the literature on this game, which gives rise to the notion of program equilibrium, see for example this blog post and the resources therein. I was introduced to it at a workshop run by the Machine Intelligence Research Institute.
We’ll call a program that plays this game a bot. The two simplest bots are CooperateBot and DefectBot, which always cooperate and always defect respectively. CooperateBot is exploitable (it gets exploited by at least one other bot), and DefectBot can never achieve mutual cooperation. Can we do better? For starters, does there exist an unexploitable bot that is not DefectBot (equivalently, that achieves mutual cooperation against at least one other bot)?
As it turns out, there is. CliqueBot is the bot that checks its opponent’s source code to see if it’s identical to its own source code (which it has access to via quining; see also Kleene’s recursion theorem). If so, it cooperates; otherwise, it defects. CliqueBot defects against every bot except CliqueBot and achieves mutual cooperation with CliqueBot, so it satisfies our requirements.
Another interesting example is FairBot (although the version I’m about to define is not the version defined in the blog post I linked to), which executes its opponent’s source code when fed its own source code as input and returns the result as its move. FairBot can be seen as trying to instantiate the symmetry argument for cooperation. However, it ends up running forever when it plays against itself, so it doesn’t in fact achieve mutual cooperation with itself. This can be fixed by modifying FairBot so that it searches for proofs in, say, Peano arithmetic of up to some fixed length that its opponent cooperates and cooperates if it finds such a proof. Somewhat surprisingly, a variant of Löb’s theorem can be used to show that this version of FairBot cooperates with itself (see the blog post previously linked to above for details).
The total prisoner’s dilemma with visible source code
To connect back to Cantor’s theorem, we will impose an additional requirement but relax a previous requirement. Namely, we will impose the additional requirement that bots are required to halt: in other words, they can’t run forever but must eventually output either cooperate or defect. However, we will relax the requirement that bots must be programs: in other words, we will allow them to potentially implement arbitrary functions. More precisely, define a bot environment to be a set of bots together with a function
describing, for each pair of bots, whether
cooperates or defects against opponent
. We place no other constraints on the set
or the function
. For example, any set of programs in the prisoner’s dilemma with visible source code which are guaranteed to halt against each other defines a bot environment.
Say that a bot in a bot environment is a CantorBot if it has the property that
; in other words, it cooperates against precisely those bots which do not cooperate with themselves.
No-CantorBot Theorem: No bot environment contains a CantorBot.
Proof. CantorBot’s move against itself is not well-defined, since its definition requires that .
Cantor’s theorem follows as a corollary. To see this we need to curry the function to obtain a function
.
By mapping , we can identify
with the power set of
, and then we can think of
as the function which describes for each bot the set of all bots it cooperates with. If
is a surjection, then there must exist bots which exhibit all possible cooperation behaviors against other bots. The above proof shows that this can’t be the case by showing that there does not exist
such that
since such an is precisely a CantorBot. (Strictly speaking, the claim that in any bot environment, not all cooperation behaviors are exhibited is the correct restatement of Cantor’s theorem in this language.)
The reasoning above is essentially the same as in the Barber paradox, and hence in Russell’s paradox, but with “ cooperates with
” in place of “
shaves
” and “
contains
” respectively. Note that in the Barber paradox and in Russell’s paradox there are extra and unnecessary assumptions placed on the “shaves” and “contains” relations; in the No-CantorBot theorem, “
cooperates with
” is an arbitrary relation on a set
.
The halting problem
One interesting observation about CantorBot is that it exists in the usual prisoner’s dilemma with visible source code: a bot receiving its opponent’s source code can attempt to compute what
would do against itself and do the opposite of that. CantorBot will, of course, run forever against many opponents, including itself, which is why the above proof doesn’t apply to this case. In other words, functions described by a program are not really functions but partial functions: they may run forever and not produce an output.
We can think of a partial function as an ordinary function
, where
is the output that corresponds to running forever. And it’s only a very small jump from here to a proof of the halting theorem. We just think of the set
of programs as a bot environment, equipped with the function
given by if
halts given
as input and
otherwise. When we run this bot environment through the proof of the No-CantorBot theorem, we get the following. Suppose the halting problem is decidable by a Turing machine. Then we can program a CantorBot which, when fed the source code of another bot
, halts if
runs forever given itself as input and runs forever if
halts given itself as input. This CantorBot can neither run forever nor halt given itself as input, and therefore doesn’t exist; contradiction.
Note that Cantor’s theorem, when applied to this situation, only claims that there exists a pattern of halting or running forever when fed various programs as input that isn’t exhibited by an actual program, which implies in particular that there exists an undecidable problem about Turing machines. But the No-CantorBot theorem actually exhibits such a problem, namely the halting problem.
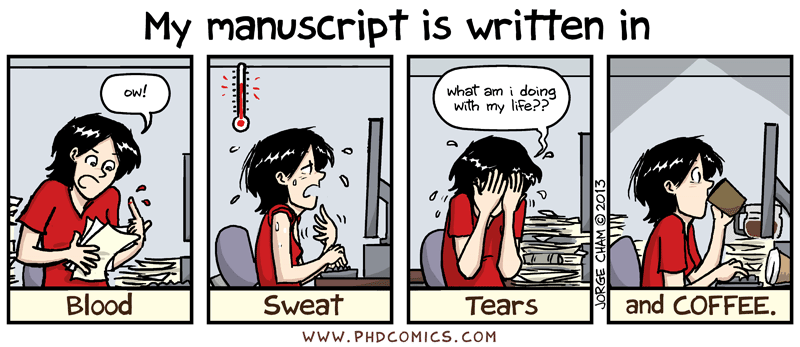
07/5/13 PHD comic: 'Written'
| Piled Higher & Deeper by Jorge Cham |
www.phdcomics.com
|
|
 |
||
|
title:
"Written" - originally published
7/5/2013
For the latest news in PHD Comics, CLICK HERE! |
||
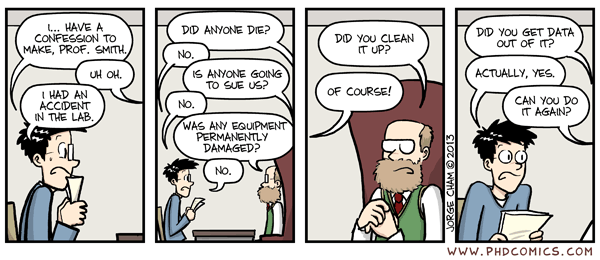
07/29/13 PHD comic: 'Confession'
| Piled Higher & Deeper by Jorge Cham |
www.phdcomics.com
|
|
 |
||
|
title:
"Confession" - originally published
7/29/2013
For the latest news in PHD Comics, CLICK HERE! |
||
Esperanto: the second language in OUYA
Since the following article is about a project I’m personally involved in, I will translate the article about it from Libera Folio, an Esperanto news source.
After English, Esperanto became the second language, which appeared in the recently launched game console OUYA, a system crowdfunded by private hobbyists. An app for listening to Esperanto radio was already released just four weeks after the device’s launch.
The small and inexpensive game console OUYA is based on the open operating system Android, and raised $8.5 million on Kickstarter. All games for the new device are free to try, but gamers can purchase extra content within games. The console itself is relatively inexpensive, being priced at $99.
The system was launched on June 25, 2013. Its interface is still only in English and all of its app are likewise in English. A month later, the first app in a language other than English appeared: Esperanto-radio Muzaiko, letting OUYA owners listen to the non-stop online Esperanto radio station Muzaiko as well as other Esperanto stations on their television set.
“I remembered, that Jacob Nordfalk wrote an app to listen to Esperanto radio on Android phones and I thought it would be easy to port it to OUYA. It was, in fact, more work than I thought, but I’m satisfied with the result! Because the code itself is open source, it was very easy to collaborate on the Google Code platform,” says Chuck Smith, who worked on this project along with Jacob Nordfalk.
Besides listening to Esperanto radio on TV, the console is mostly used for video games. It’s also possible to unofficially install other Android apps on it, but you’re never sure if they’ll work, since Android apps are typically made for touchscreens, while a game controller is used to interact with this device.
For more details: English press release
You can also listen to Muzaiko on other devices, like your computer.
sweet-land-of-libertea: thackerybinxx: shinga-tumblr: It’s...




It’s okay kitten, I too have been that drunk
OH my GOD
infomercial kitten.
why is no-one willing to sell him a special kitten straw for $19.95
Masturbação feminina agora tem aplicativo
É fato que a masturbação é tabu em muitas culturas, devido a fatores diversos, como a religião, mas também é fato que é preciso conhecer o próprio corpo, saber como ele funciona e responde a determinados estímulos. A masturbação feminina é ainda um tabú maior do que a masculina, e para tentar quebrar um pouco do preconceito, a designer americana Tina Gong, também conhecida como Milkmusket, desenvolveu um aplicativo que não só dá informações sobre o assunto, mas também ensina as mulheres a conhecerem seu próprio corpo em busca do prazer individual. O HappyPlayTime ainda está em desenvolvimento, mas já está dando o que falar. Confiram abaixo nas imagens:


Fonte: Fubiz